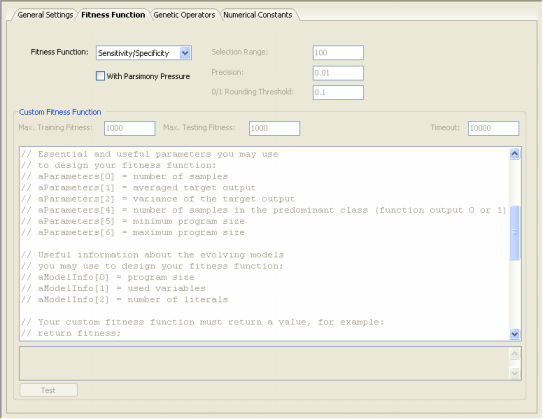
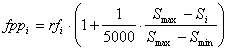| GeneXproTools 4.0 implements the Correlation
Coefficient fitness function both with and
without parsimony pressure. The
version with parsimony
pressure puts a little pressure on the size of the evolving
solutions, allowing the discovery of more compact models.
The Correlation Coefficient fitness function of GeneXproTools 4.0
is, as expected, based on the standard correlation
coefficient, which is a dimensionless index that ranges from -1 to 1 and reflects the extent of a linear relationship between
the predicted values and the target values.
where Cov(T,P) is the covariance of the target and model outputs; and st and sp are the corresponding standard deviations, which are given by:
where P(ij) is the value predicted by the individual program
i for sample case j (out of n fitness cases
or sample cases);
Tj is the target value for fitness case j; and
The correlation coefficient is confined to the range [-1, 1]. When
Ci = 1, there is a perfect positive linear correlation between
T and P, that is, they vary by the same amount. When Ci = -1, there is a perfect negative linear correlation between
T and P, that is, they vary in opposite ways (when T increases,
P decreases by the same amount). When Ci = 0, there is no correlation between
T and P. Intermediate values describe partial correlations and the closer to 1 or -1 the better the model. fi = 1000*Ci*Ci and therefore ranges from 0 to 1000, with 1000 corresponding to the ideal.
where Si is the size of the program, Smax and Smin represent, respectively, maximum and minimum program sizes and are evaluated by the formulas: Smax = G (h + t) Smin = G where G is the number of genes, and h and t are the head and tail sizes (note that, for simplicity, the linking function was not taken into account). Thus, when rfi = rfmax and Si = Smin (highly improbable, though, as this can only happen for very simple functions as this means that all the sub-ETs are composed of just one node), fppi = fppmax, with fppmax evaluated by the formula:
|